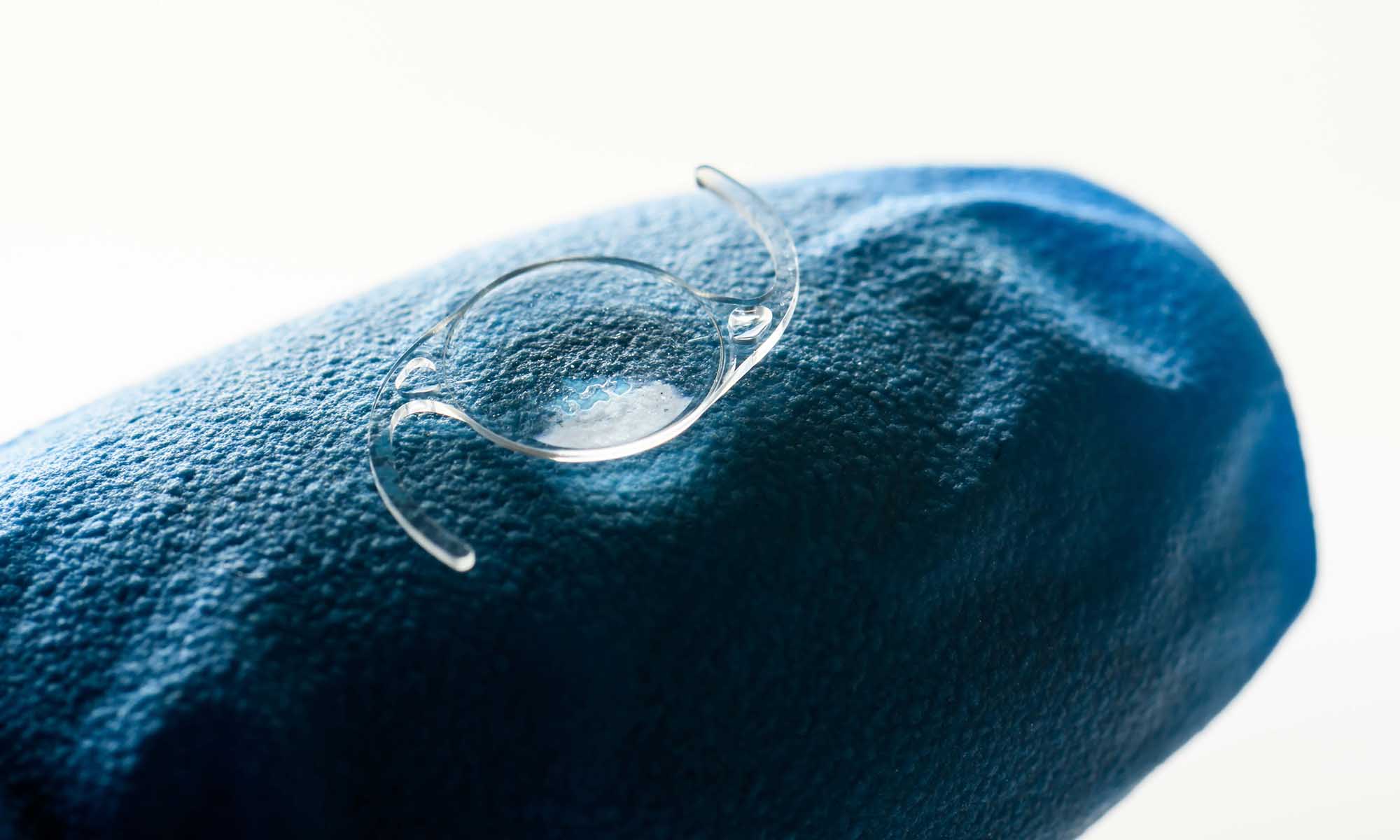
Science & Technology
What are intraocular lenses? And how can they restore sight for patients with cataracts?
New technology is quickly improving the world’s most common form of eye surgery.

Rochester researchers on the growing concern of plastics exposure on human health.